Category Archives: Maths Challenges

View post →

View post →

Trigonometry is a really interesting branch of mathematics which is all about triangles and their angles.
If you are lucky enough to have a scientific calculator, you could experiment with it to find out about the relationships between the length of different sides of triangles (and different types of triangles) and the sines, cosines and tangents of all the angles.

e.g. If I had an equilateral triangle, all the sides would be equal (let’s say they are all 6 cm). In this case, the cosine of the base angles would be found by dividing half of the baseline (= 3 cm) by the (hypotenuse) side that we know is 6 cm. So we have Cosine of the base angle = 3/6 = 0.5. So inverse Cosine 0.5 is the angle at the base of the triangle… Look it up to see what you get…

There is also a useful site available on ‘Maths is fun’ which will introduce you to the ideas if you want to look further… Click on the image above to follow the link and play with the angles to find out more.

View post →

Here is the other NRICH Countdown game we tried last week. There are several levels to this game which do get harder. We were managing Levels 1 and 2 pretty well on Friday. Maybe we could try out Level 3? See what you think of it.

Here is the link to the NRICH countdown game we played last week.

Click on the image to go to the online game.

This is a fun way to practise your mental maths and apply your number skills.

We had a fun maths lesson on Friday which has left us all thinking!

We started with oblong pieces of paper (A5) and the challenge was to see how many 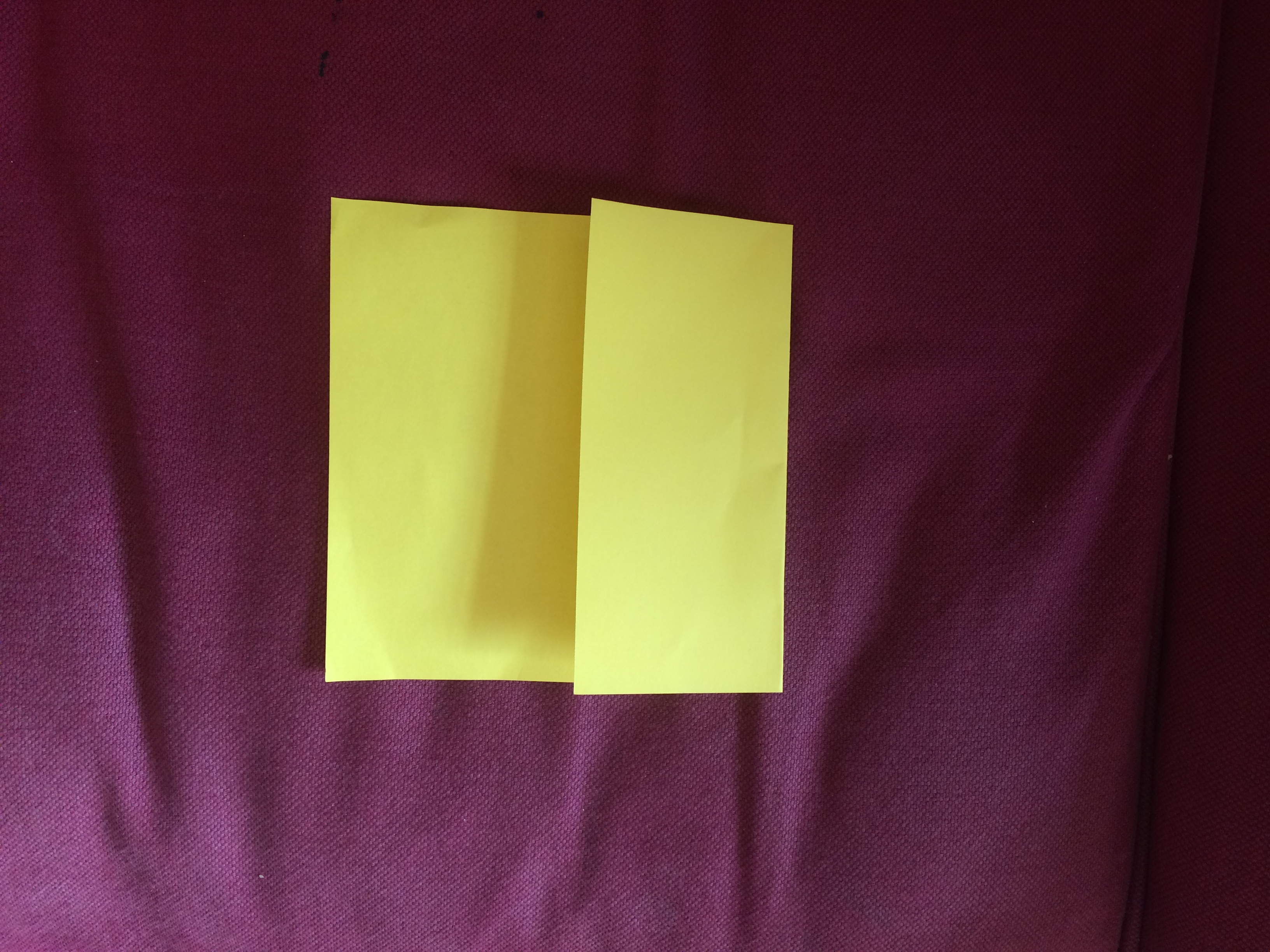different polygons we could make using one fold only. We then had to explore the properties of the different shapes and look for symmetry, regularity, etc. We discovered that we could not make a triangle with only one fold, but we could make a variety of quadrilaterals (discussed square (regular quadrilateral), trapezium, creating different parallelograms, etc.).

Once we started looking at higher order polygons, we discovered we could make shapes up to nonagons but none were regular and only the shapes with odd numbers of sides (pentagon, heptagon, nonagon) could be created to have a line of symmetry with one fold.

Next someone suggested seeing how many different polygons they could make with two folds. Some people insisted they could make a tetradecagon (14), but others thought they could only make 13 (triskaidecagon). We are not entirely sure whether we are folding accurately so it would be good to try this again and confirm which is correct.

We decided then that we would spend the weekend investigating this further to see if there is a pattern that we can come up with to predict the maximum number of sides of polygons that can be made with a given number of folds and whether there is a mathematical explanation for this. We are sure there must be but we think we might need help to find out. So far, we think we have a sequence of 1 fold (max 9 side), 2 folds (max 13 sides), 3 folds (17 sides) which looks like there could be a pattern developing but it is getting harder and harder to be sure we have folded correctly which is why we think it would be good to see what other people think. We are wondering whether the increase in 4 each time has also got something to do with the number of sides in our initial shape (the oblong paper). Maybe we could investigate this further using a different polygon to start us off?

I have asked our friends in Year 6 at Long Itchington to see if they can help and am also asking some other people who love maths problems. I’ll let you know if we find out! If you discover anything more, it would be great to add a comment. There is a gold card for anyone coming up with ideas that they can explain to me clearly! We want to crack this before the end of term!